Free Energy is the Energy Available for Work
Primary tabs
Free Energy is the Cell's Available Energy
Free energy is energy available to do work.
- It can be considered the analog of potential energy in a "thermal environment" (where molecular collisions substantially alter the potential and/or kinetic energies of objects of interest - i.e., proteins, solvent, etc.).
- Systems will move from a condition of high to low free energy if it is possible: a ball will roll downhill in the absence of a barrier.
- The cell stores free energy in two primary ways:
- (1) a gradient - i.e., differing concentrations of ions or small molecules across a membrane, as is the case for some transporters.
- (2) activated carriers - i.e., molecules capable of dissociating, but which are maintained at a concentration well above the equilibrium value, such as ATP and GTP.
- Any system that is out of equilibrium stores free energy that can be used for work - e.g., to drive cellular processes such as transport, locomotion, synthesis - or signaling processes. Conversely, an equilibrium system stores no free/usable energy unless the conditions are changed.
Activated Carriers Store Free Energy

The cell maintains the concentration of an activated carrier (e.g., GTP or ATP) well above its equilibrium value so that there is always a driving force toward equilibrium (via the decomposition reaction). This phenomenon is naturally quantified using the chemical potential. Because there is a drive toward equilibrium, there is free energy which can be harnessed for work - as in the example of ATP-driven transporters. The free energy stored in GTP is used by the translation machinery (ribosome, etc.) to increase protein fidelity to the mRNA sequence in a process called "kinetic proofreading".
Concentration Gradients across Membranes Store Free Energy

As in the case of activated carriers, gradients typically are also out of equilibrium: there is a driving force to equalize the concentrations of species across a membrane (assuming for simplicity no coupling among species). A more quantitative discussion of this phenomenon is available; see also the discussion of the chemical potential. The cell can use gradient-stored free energy, for instance in the case of active transport.
The Cell Uses Free Energy in Different Ways
In general, the cell uses free energy it stores in gradients and activated carriers to accomplish two types of things:
- (1) Work in the usual sense - i.e., energetically unfavorable tasks such as transport (against a gradient), locomotion, and chemical synthesis.
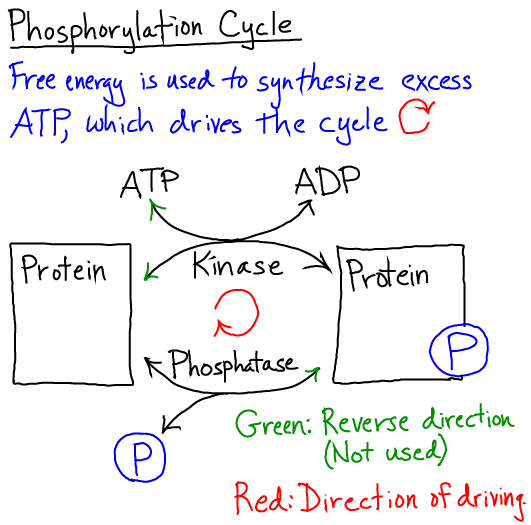
- (2) Energy-neutral signaling processes, such as phosphorylation, which involve a specific sequence of events that may leave the signal carriers (e.g., proteins) unchanged.
How does the free energy remain stored without "running downhill"?
Free energy in the cell will not dissipate unless the transition from the high-free-energy non-equilibrium state toward a lower free-energy state is catalyzed: for activated carriers, enzymes are required; for gradients across membranes, flow is enabled by channels or transporters.
How does the cell get energy?
The cell maintains its supply of ATP and other stored energy by metabolizing nutrients in an ongoing cycle of ATP synthesis.
Cycles and the Cell's Non-equilibrium Use of Free Energy

Cellular processes typically function in cycles to re-use molecular components, and free energy is used to drive such cycles in a single direction by maintaining some components out of equilibrium - e.g., supplying excess ATP or other substrates for catalysis.
Conformational Free Energy
Biomolecules and biomolecular complexes also work with conformational free energy, which can be used for transient energy storage during a multi-step process, as seen in the antiporter and ATP synthase examples. Discussion of the microscopic origins of conformational free energy is given in the page on conformational statistical mechanics.
