Primary tabs
Chemical Reactions: Catalysis
Essentials of Catalysis (Enzyme Action)
Key points
- Catalysis is the acceleration of a molecular process, such as a chemical reaction (i.e., covalent change), isomerization (i.e., conformational change), or a binding process. The catalyst remains unchanged after the process.
- It goes both ways: All molecular processes are reversible, and a given catalyst (e.g., an enzyme) necessarily catalyzes both directions of the reaction equally well, as explained below. This key point is sometimes overlooked.
- In the cell: Very few chemical reactions necessary for cellular activity (e.g., phosophorylation, chemical synthesis) occur spontaneously during the lifetime of a cell. This necessitates catalysis, but more importantly, provides a means for the cell to regulate its processes. In essence, reactions only happen when the necessary enzyme is present and active, and the presence/activity of enzymes is tightly regulated through control of protein expression, degradation, and post-translational modifications such as phosphorylation.
Basic Catalysis: Isomerization or Unimolecular Chemical Change
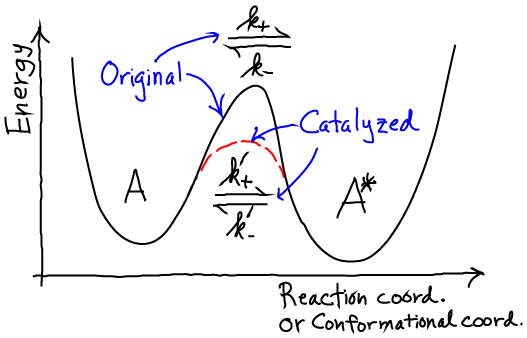
- Isomerization is a conformational change in a small molecule or a macromolecule like protein, RNA, or DNA.
- A unimolecular reaction is one affecting only a single molecule, perhaps by forming or breaking a single covalent bond within the molecule.
- Either situation can be schematized using a simple energy landscape, in which high energy barriers are rarely overcome (i.e., characterized by low rates).
In catalysis, rates increase. Denoting catalyzed rates with primes, we have
 (1)
(1)However, catalysis cannot change the equilibrium ratio of the state populations $\conc{A} / \conc{A*}$. Mathematically,

In words, the catalyzed rates increase together so as to maintain the proper equilibrium - which is unaffected by the presence of a catalyst.
There is a nice way to convince yourself that catalysis cannot change the equilibrium point - it is a worthwhile exercise. Consider a cycle in which the two states of interest are connected by both a catalyzed and uncatalyzed process. You can show that, if the ratio of forward and reverse rates does not agree for the two cases, the cycle will spontaneously circulate - violating the second law of thermodynamics and enabling the construction of (imaginary) energy-creating processes.
Chemical Reactions: Catalysis
Essentials of Catalysis (Enzyme Action)
Key points
- Catalysis is the acceleration of a molecular process, such as a chemical reaction (i.e., covalent change), isomerization (i.e., conformational change), or a binding process. The catalyst remains unchanged after the process.
- It goes both ways: All molecular processes are reversible, and a given catalyst (e.g., an enzyme) necessarily catalyzes both directions of the reaction equally well, as explained below. This key point is sometimes overlooked.
- In the cell: Very few chemical reactions necessary for cellular activity (e.g., phosophorylation, chemical synthesis) occur spontaneously during the lifetime of a cell. This necessitates catalysis, but more importantly, provides a means for the cell to regulate its processes. In essence, reactions only happen when the necessary enzyme is present and active, and the presence/activity of enzymes is tightly regulated through control of protein expression, degradation, and post-translational modifications such as phosphorylation.
Basic Catalysis: Isomerization or Unimolecular Chemical Change

- Isomerization is a conformational change in a small molecule or a macromolecule like protein, RNA, or DNA.
- A unimolecular reaction is one affecting only a single molecule, perhaps by forming or breaking a single covalent bond within the molecule.
- Either situation can be schematized using a simple energy landscape, in which high energy barriers are rarely overcome (i.e., characterized by low rates).
In catalysis, rates increase. Denoting catalyzed rates with primes, we have
 (1)
(1)However, catalysis cannot change the equilibrium ratio of the state populations $\conc{A} / \conc{A*}$. Mathematically,

In words, the catalyzed rates increase together so as to maintain the proper equilibrium - which is unaffected by the presence of a catalyst.
There is a nice way to convince yourself that catalysis cannot change the equilibrium point - it is a worthwhile exercise. Consider a cycle in which the two states of interest are connected by both a catalyzed and uncatalyzed process. You can show that, if the ratio of forward and reverse rates does not agree for the two cases, the cycle will spontaneously circulate - violating the second law of thermodynamics and enabling the construction of (imaginary) energy-creating processes.
